IntroductionScanning Force Microscopy (SFM)

The scanning force microscopy (SFM), also known as atomic force microscopy (AFM), belongs to the branch of scanning probe microscopy (SPM), which comprises all microscopy techniques that form pictures of surfaces not by optical or electron-optical imaging, but due to interaction of a physical probe with the sample.

The precursor to the SFM, the scanning tunneling microscope (STM), was developed by Gerd Binnig and Heinrich Rohrer in the early 1980s [1, 2] und earned them the Nobel Prize for Physics in 1986. The first SFM was invented by Binnig, Quate and Gerber in 1986 [3]. Its alternative name, AFM, refers to the interactions between probe and sample on the atomic level. The attractive van-der-Waals forces and the Pauli repulsion due to overlapping electron orbitals can be described by the Lennard-Jones-potential. 

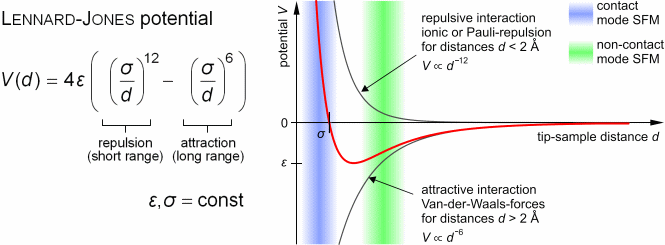(Figure by Steve Pawlizak, 2009.)

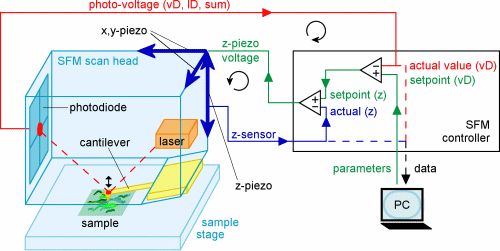	(Figure taken from diploma thesis of Claudia Brunner, 2004.)

Since an SFM can image and probe samples in both dry and liquid environments, it is possible to work with living cells under physiological conditions [4, 5]. For our research, we are using the NanoWizard BioAFM (JPK Instruments AG, Berlin), where the x-y-z-scanner is attached to the cantilever mount (see figure). The NanoWizard has the great advantage of using a variety of light microscopy techniques (e.g. phase contrast) together with the SFM-technique, which is especially helpful for biological research.
 

Imaging Modes

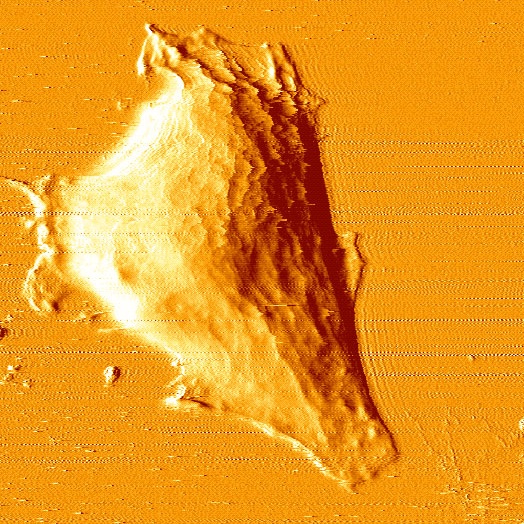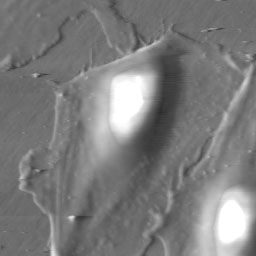
An SFM image scan visualizes the topography of the surface and can be used to create a three dimensional representation.
There are different imaging modes for SFM, primarily distinguished into static mode (contact mode) and dynamic modes (non-contact and intermittent contact mode) with oscillating probe. The two most commonly used modes for imaging biological samples are the contact and the intermittent contact mode (tapping mode). While in contact mode the tip scratches the surface of the sample, the cantilever in intermittent contact mode vibrates and thus taps point after point of the sample.
 

Force Spectroscopy
 
 

	(Figure taken from diploma thesis of Steve Pawlizak, 2009.)

Besides imaging, there is another important application of SFM. That is force spectroscopy. In this mode, the cantilever is pushed into the sample at a certain point and subsequently retracted. During this process the height information of the z-piezo element and the vertical deflection of the cantilever detected by the four-quadrant photodiode are recorded. The result is a so-called force-distance-curve (since the deflection is closely related to the force that the tip applies to the sample). To be more precise, each scan produces two curves, one showing the cantilever deflection u in dependence on the height z while approaching the sample (trace curve) and another one for the retraction (retrace curve). On a quasi-infinitely hard substrate (e.g. glass coverslip), a characteristic graph is produced, which is discussed in the following.
Trace: First the cantilever is moved down without touching the sample, i.e. no deflection but a declining distance is measured (1). Very close to the surface the cantilever can be suddenly attracted by the sample due to adhesion forces (e.g. electrostatic interaction), i.e. the cantilever flicks down the remaining distance and gives a small downward deflection (2). When the cantilever is moved further down, the cantilever is bent upwards in direct proportion to the z-piezo height (3). This characteristic linear slope can be used for calibration of the cantilever.
Retrace: As soon as a defined setpoint of deflection is reached (4), the cantilever is withdrawn. The cantilever gets more and more unbent, while moving upwards again (5). Then, the tip usually keeps attracted to the surface by adhesion, which causes the cantilever to bend in the opposite direction, until it suddenly loses contact and flicks up into its initial position (6). Further retraction results no longer in a vertical deflection (7).

When the cantilever is calibrated, i.e. its sensitivity s and spring constant k are know, it is possible to calculate the applied force F which is proportional to the vertical cantilever deflection u.

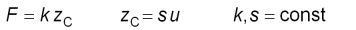
Measurement of Elastic and Viscoelastic Properties
 
 

	(Figure by Claudia Brunner and Steve Pawlizak, 2004, 2009.)

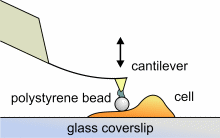
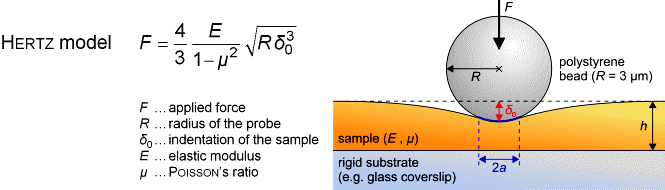
SFM may be used to measure local elastic and viscoelastic properies of soft matter samples like biological cells. The local elastic modulus E can be determined by recording and analyzing force-distance-curves. In order to avoid damages of living cells during the measurement and to have well-defined probe geometry for the following calculation of the moduli, we modify commercially available cantilevers by gluing a small polysterene bead (diameter ~ 6 µm) onto the tip. The appropriate calculations are done based on the Hertz model [6].
Dynamic SFM measurements with a vibrating cantilever can be performed to quantify even the viscous properties of the sample by determining its storage and loss modulus. In this case a modified Hertz model is used for data analysis as described in [7].
When thinner samples are to be probed, the influence of the underlying hard substrate on the elasticity measurement cannot be neglected anymore and we apply Tu and Chen corrections to the Hertz model [8]. 

(Figure by Steve Pawlizak, 2009.)

SFM can be used to measure cell-cell and cell-substrate adhesion forces by pulling on adhered cells with a functionalized cantilever. In our lab, we are using the CellHesion 200 (JPK Instruments AG, Berlin) for cell adhesion measurements.

A reduced adhesive strength is found in cancer cells which may be correlated with their potential to metastasize. We will investigate the adhesion of normal fibroblasts as well as fibroblasts throughout the progression of malignant transformation. Precise measurements of the changes in adhesive strength of malignant cells and of the correlated cytoskeletal properties will provide new insight into the role of cell adhesion in cancer metastasis.

References:
 

[1]		G. Binnig, H. Rohrer, C. Gerber, E. Weibel:Tunneling through a Controllable Vacuum Gap, Appl. Phys. Lett. 40(2):178-180 (1982).
[2]		G. Binnig, H. Rohrer, C. Gerber, E. Weibel: Surface Studies by Scanning Tunneling Microscopy, Phys. Rev. Lett. 49(1):57-61 (1982).
[3]		G. Binnig, C. F. Quate, C. Gerber: Atomic Force Microscope, Phys. Rev. Lett. 56(9):930-933 (1986).
[4]		M. Radmacher, R. W. Tillmann, M. Fritz, H. E. Gaub: From Molecules to Cells: Imaging Soft Samples with the Atomic Force Microscope, Science 257(5078):1900-1905 (1992).
[5]		F. Moreno-Herrero, J. Colchero, J. Gómez-Herrero, A.M. Baró: Atomic force microscopy contact, tapping, and jumping modes for imaging biological samples in liquids, Phys. Rev. E 69(3):031915 (2004).
[6]		H. Hertz: Über die Berührung fester elastischer Körper, Journal für die reine und angewandte Mathematik 92:156-171 (1881). PDF
[7]		R. E. Mahaffy, C. K. Shih, F. C. MacKintosh, J. Käs: Scanning probe-based frequency-dependent microrheology of polymer gels and biological cells, Phys. Rev. Lett. 85(4):880-883 (2000). PDF
[8]		R. E. Mahaffy, S. Park, E. Gerde, J. Käs, C. K. Shih: Quantitative analysis of the viscoelastic properties in thin regions of fibroblasts using AFM, Biophys. J. 86(3):1777-1793 (2004). PDF