

Over a thousand years ago, windmills were in operation in Persia and China, see TelosNet and Wikipedia. Post mills appeared in Europe in the twelfth century, and by the end of the thirteenth century the tower mill, on which only the timber cap rotated rather than the whole body of the mill, had been introduced. In the United States, the development of the water-pumping windmill was a major factor in allowing farming and ranching across vast areas in the mid-nineteenth century. These windpumps (sometimes called Western mills) are still common in America and Australia. They have a rotor with about 30 vanes (or blades) and the ability to turn themselves slowly. Of the 200,000 windmills existing in Europe in the mid-nineteenth century, only one in ten remained a century later. The old windmills have since been replaced by steam and internal combustion engines. However, since the end of the last century the number of wind turbines is growing steadily, and they are beginning to take an important role in power generation in many countries.
We first show that for all wind turbines, wind power is proportional to wind speed cubed. Wind energy is the kinetic energy of the moving air. The kinetic energy of a mass m with the velocity v is
The air mass m can be determined from the air density ρ and the air volume V according to
Then,
Power is energy divided by time. We consider a small time, Δt, in which the air particles travel a distance s = v Δt to flow through. We multiply the distance with the rotor area of the wind turbine, A, resulting in a volume of
which drives the wind turbine for the small period of time. Then the wind power is given as
The wind power increases with the cube of the wind speed. In other words: doubling the wind speed gives eight times the wind power. Therefore, the selection of a "windy" location is very important for a wind turbine.
The effective usable wind power is less than indicated by the above equation. The wind speed behind the wind turbine can not be zero, since no air could follow. Therefore, only a part of the kinetic energy can be extracted. Consider the following picture:
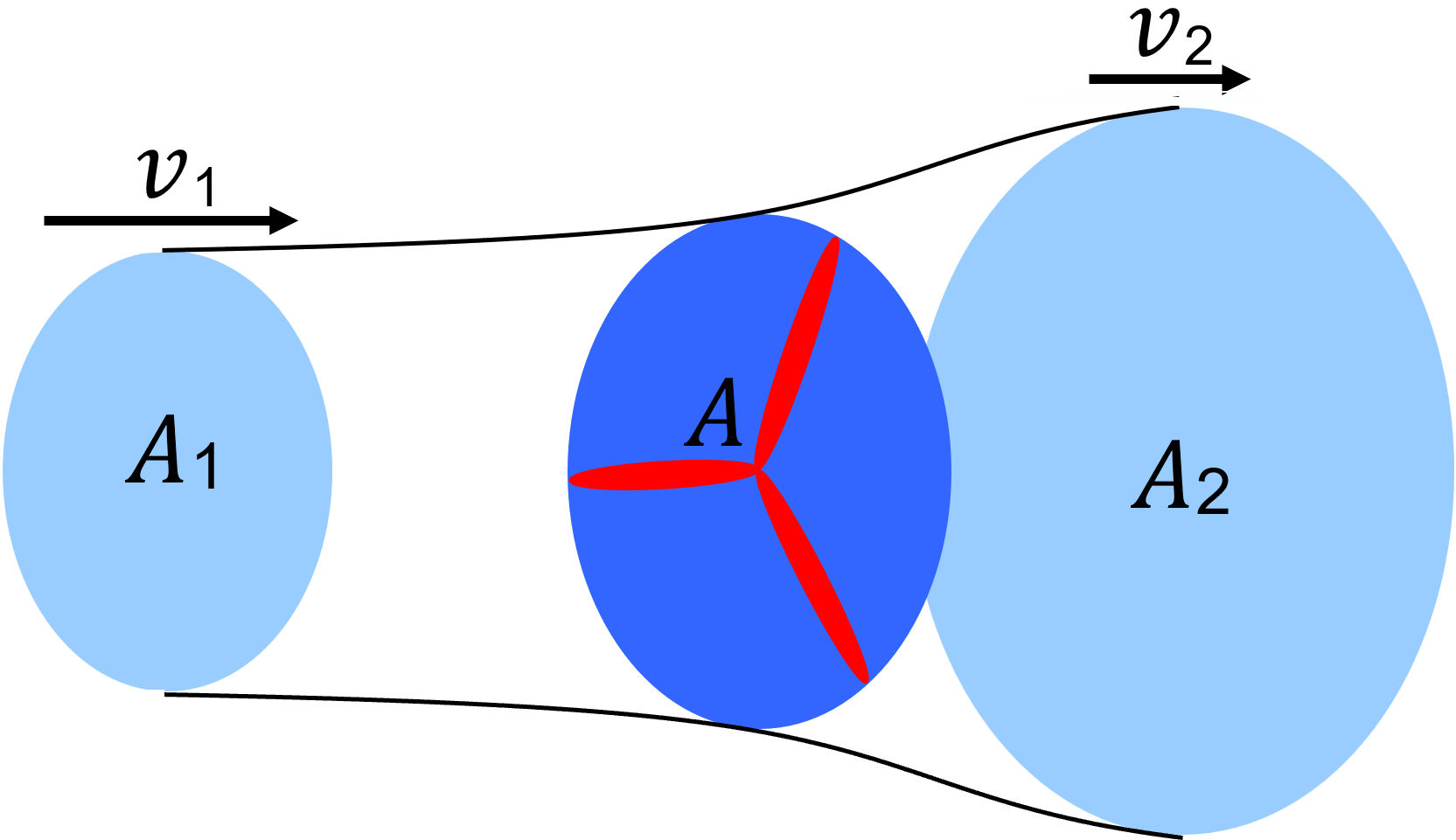
The wind speed before the wind turbine is larger than after. Because the mass flow must be continuous, A v = constant, the area A2 after the wind turbine is bigger than the area A1 before. The effective power is the difference between the two wind powers:
If the difference of both speeds is zero, we have no net efficiency. If the difference is too big, the air flow through the rotor is hindered too much. The power coefficient cp characterizes the relative drawing power:
To derive the above equation, the following was assumed: A1v1 = A2v2 = A (v1+v2) / 2. We designate the ratio v2/v1 on the right side of the equation with x. To find the value of x that gives the maximum value of CP, we take the derivative with respect to x and set it to zero. This gives a maximum when x = 1/3. Maximum drawing power is then obtained for v2 = v1 / 3, and the ideal power coefficient is given by
Another wind turbine located too close behind would be driven only by slower air. Therefore, wind farms in the prevailing wind direction need a minimum distance of eight times the rotor diameter. The usual diameter of new wind turbines is 140 m with an installed capacity of 5 MW onshore. 200 m diameter and 10 MW will come soon offshore.
The installed capacity or rated power of a wind turbine corresponds to an electrical power output of a speed between 12 and 16 m/s, with optimal wind conditions. For safety reasons, the plant does not produce greater power at the high wind conditions than those for which it is designed. During storms, the plant is switched off. Throughout the year, a workload of 23% can be reached inland. This increases to 28% on the coast and 43% offshore.
More details can be found in the Internet pages wind-works.org and in the pages of the American Clean Power Association (ACP).
For 2023, IRENA reports an installed wind power capacity of 1017 GW worldwide and 148 GW in the United States. The Alta Wind Energy Center in California has been the largest wind farm in the United States since 2013, with a capacity of 1.6 GW. The electricity produced from wind power in the United States amounts about 10% of all generated electrical energy. Detailed information about the present state in the US can be found in Wikipedia and in a 2024 PNNL report.
A crucial point about wind power is that the times of peak electricity demand and the times of optimal wind conditions rarely coincide. Thus, other electric power producers with short lead times and a well developed electricity distribution system are necessary to supplement wind power generation.
Why have the wind turbines of today lost one blade in comparison to the old four-blade windmills?
The rotor power Pmech = 2π M n is proportional to the torque M acting on
the shaft and the rotation frequency n. The latter is influenced by the tip speed ratio λ,
which is calculated according to λ = vu / v1 from the ratio of
peripheral speed (tip speed) vu of the rotor and the wind speed v1.
The torque M increases with the number of blades. It is therefore largest for the many-vaned Western mills,
smaller for wind mills with four blades, and smallest for today’s wind turbines with 3 blades. However, every blade,
as it rotates, reduces the wind speed for the following blades. This "wind shadow" effect increases with the number of blades.
The optimal tip speed ratio is about one for the Western mill, barely over 2 for the four-bladed type, and 7−8 for
the three-bladed rotors. At their optimal tip speed ratio, three-bladed rotors achieve a cp value
of 48% and come closer to the ideal value of 59% than wind turbines with 4 blades.
For wind turbines with two blades or weight-balanced one-bladed rotor configurations, the yield is smaller in spite of
a higher tip speed ratio, because of the smaller torque M. Therefore, wind turbines today have three blades.